Random Gem Generation

In general, I like tabletop RPG rules to be lightweight and quick.  But, like most people, I have a few spots that I enjoy getting carried away in.  For me, gemology has always been fascinating, so I like to have some extra variation in them beyond "You find a 100gp Gem!".  Here's what I have done for Phoenix.

Oooooh shiny!

In the spirit of keeping the system lightweight, I limited myself to no more than three primary dice rolls.  This was challenging, but I would otherwise get lost in tables of inclusions, occlusions, styles of cut, luminescence, etc.  The 3-roll rule forced me to prioritize and use abstractions.  I did let myself use "subrolls" - up to one level deep - to provide some additional variation.  These subrolls were not allowed to consult additional tables.

An important requirement was that the system had to scale by tier, but without separate tables.  So, I constructed my base gem table so that it would use Tier Dice (dT).  In the current draft, that's 2d10 for Mortals, 3d10 for Heroes, and 4d10 for Demigods.  Since d10's always explode in Phoenix, that also means that every so often a Mortal could get an amazing gem in their treasure - that's ok for me, as I like the drama such results can drive.

I use the silver standard in these tables, but you can change it to any suitable currency.  In my rules, 1gp = 100sp.

Here is roll #1.

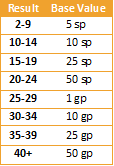Base Gem Values (dT)

While constructing the above table, I spent some quality time with AnyDice, working out exploding dice probabilities to make sure I got the results I wanted.  Here's a table summarizing the statistics.

Gem Statistics

Mode represents the most common result for a given tier, and Mean represents the average value of all rolls.  As you can see, the variance between these values increases dramatically by tier - exactly what I wanted.  Over time, a demigod will average 6gp, but they will get a wide variety of gems from the cheap to the expensive.  On the other hand, a mortal will almost always get cheap gems.

I wanted the mean to hold true, allowing GMs to come up with any sort of system they desired, so an additional objective was that rolls 2 and 3 should net out to the same mean - they should create flavor, and possibly variance, but not alter the average value of the gems.

For roll #2, I decided on weight.  I've always wanted to use carats as my gem measurement in RPGs, so it was a natural choice.  The values in roll #1 are the per-carat values for each gem class.

Trivia:  A carat is equal to 200mg (or 0.007055 ounces for us silly Americans), and its relation to size depends on the specific gravity of the gem.  Specific gravity is the density of a gem, as compared to water.  A larger specific gravity means the gem is more dense.  Amber has a very low specific gravity (~1), and rubies have a very high specific gravity (~4), so a 1 carat amber is quite a bit larger than a 1 carat ruby!  GemSelect has a great chart of specific gravities, if you are curious about that level of descriptive detail.

Random Gem Weights (d%)

This table is deceptively simple, thanks to the exploding d10 and d6 subrolls.  The smallest gem is one-tenth of a carat, and I've set a rule in Phoenix that any gem smaller than one-tenth of a carat is considered powder - if only the mall jewelry stores would do the same!  Despite the small chances of a large gem, this table actually produces a net increase in values for the gems, by about 15%.  This means the next roll needs to reduce our running total.

Trivia:  How realistic is this weight variance?  For small gems, pretty decent.  For large gems, not really - the largest diamond on Earth is 545.67 carats (the Golden Jubilee).  That's technically achievable with roll #2, but with a 1d6 exploding, you'd have to roll a six 90 times in a row - and then get a 5.  That will happen about once every 10⁷¹ rolls.  Sort of the same odds as picking a specific random atom, given a bag containing all the atoms in the universe (give or take a galactic cluster).

Finally, roll #3.  It had to be quality - and it had to not require a gemology degree to comprehend.  I kept it simple.

Random Gem Quality (d%)

The exact subrolls and probabilities were engineered to produce a complement to the +15% that roll #2 created, and they do so successfully - given an infinite number of rolls, using rolls 2 and 3 together vary the value of the base gem rolls by less than 1%.

All we need now are some examples of gem names for each base value class.

Gems, categorized by value-per-carat

Values are usually relative to yield, so these might vary in your own campaign world - having dwarves and other subterranean cultures mining extensively could mean that emeralds are much more common - or perhaps white quartz is more valuable than diamonds.  Maybe you have a gem called Ixilite.  It's fantasy, live a little.

Using the above tables, here's 5 random gems for each tier.

Mortal Gems
1ct White Quartz, worth 5sp
0.7ct Bloodstone, worth 7sp
0.8ct uncut Blue Zircon, worth 21sp
0.7ct powdered Bone, worth 1sp
0.1ct poor Bloodstone, worth 1sp

Heroic Gems
4ct Red Coral, worth 1gp
0.8ct poor Eye Agate, worth 3sp
0.6ct Red Zircon, worth 30sp
0.2ct powdered Blue Topaz, worth 1sp
0.7ct Pyrite, worth 4sp

Demigod Gems
1ct Beryl, worth 1gp
0.9ct Purple Zircon, worth 9gp
0.9ct remarkable Bloodstone, worth 11sp
0.3ct uncut Red Zircon, worth 3sp
4ct poor Peridot, worth 88sp

This system isn't the most comprehensive random gem system ever made, but I feel that it meets my desire for variation while also having a predictable baseline.

I know this was a long post - if you made it this far, cheers!